In this paper we use non-polynomial spline functions to develop numerical methods to approximate the solution of 2nd kind Volterra integral equations. Numerical examples are presented to illustrate the applications of these method, and to compare the computed results with other known methods.

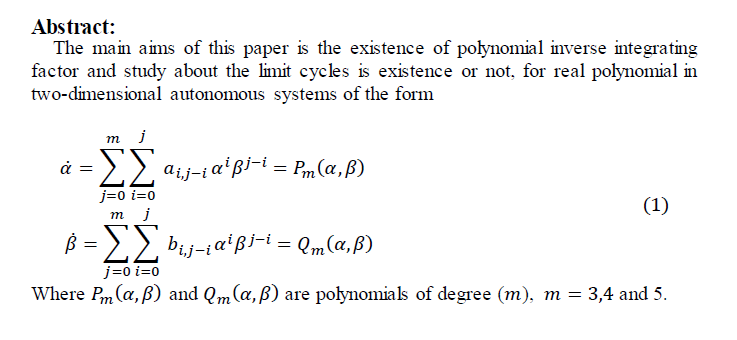
Abstract

This study aims at investigating the relationship between mindfulness and academic self-efficacy among Northern Border University students. To achieve this objective, the researcher adopted the correlative survey method for (97) students. For data collection, the researcher developed a mindfulness scale consisting of (42) items divided into seven topics, each one consisting of six items. The researcher developed an academic self-efficacy scale consisting of (20) items, adopting a five-point Likert scale. The results showed that there is a high level of mindfulness among students at the level of the seven units which formed the mindfulness scale; the conscious thinking unit showed the highest mean value o

The inverse kinematics of redundant manipulators has infinite solutions by using conventional methods, so that, this work presents applicability of intelligent tool (artificial neural network ANN) for finding one desired solution from these solutions. The inverse analysis and trajectory planning of a three link redundant planar robot have been studied in this work using a proposed dual neural networks model (DNNM), which shows a predictable time decreasing in the training session. The effect of the number of the training sets on the DNNM output and the number of NN layers have been studied. Several trajectories have been implemented using point to point trajectory planning algorithm with DNNM and the result shows good accuracy of the end

A method for Approximated evaluation of linear functional differential equations is described. where a function approximation as a linear combination of a set of orthogonal basis functions which are chebyshev functions .The coefficients of the approximation are determined by (least square and Galerkin’s) methods. The property of chebyshev polynomials leads to good results , which are demonstrated with examples.

The research entitled: (The Constructive Mutation of installation Systems in the Artworks of the artist Ali Al-Najar) has dealt with the concept of Mutation and its systematizations in installation in the artworks of (Ali Al-Najjar).
The research has four chapters: The first Chapter deals with the methodological framework represented by the basic problem of the research, that is concerned with the constructive mutation of installation systems.
The research aims at finding out the constructive mutation of installation systems in the artwork of ( Ali al-Najar). The research is limited by analyzing visual samples of (Ali Al-Najjar) artworks betwen (1967-1991)
The second chapter deals with the theoretical framework, it has five s

The paper aims at initiating and exploring the concept of extended metric known as the Strong Altering JS-metric, a stronger version of the Altering JS-metric. The interrelation of Strong Altering JS-metric with the b-metric and dislocated metric has been analyzed and some examples have been provided. Certain theorems on fixed points for expansive self-mappings in the setting of complete Strong Altering JS-metric space have also been discussed.

Background: The objective of this in vitro study was to evaluate the vertical marginal fit of crowns fabricated with ZrO2 CAD/CAM, before and after porcelain firing cycles and after glaze cycles. Materials and Methods: An acrylic resin model of a left maxillary first molar was prepared and duplicated to have Nickel-Chromium master die. Ten die stone dies were sent to the CAD/CAM (Amann Girrbach) for crowns fabrication. Marginal gaps along vertical planes were measured at four indentations at the (mid mesial, mid distal, mid buccal, mid palatal) before (Time 0) and after porcelain firing cycles (Time 1) and after glaze cycles (Time 2) using a light microscope at a magnification of ×100. One way ANOVA LSD tests were performed to determine wh